The Race of Multi-Tasking Clowns

Many contestants entered the unicycle race, but only the best multi-tasking clown came out on top, considering that each had to juggle clubs while trying to win the race! Most of the pack were soon disqualified after dropping a club or falling off the unicycle. In the end, four of the best clowns crossed the finish line. 

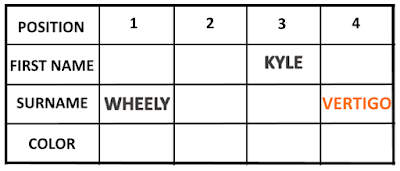
From this information and the clues below, can you determine each clown's full (real) name, club color (one is silver), and placement?

Places: 1st, 2nd, 3rd, 4th
First Names: Kyle, Matt, Jake, Leon
Surnames: Turner, Pettle, Vertigo, Wheeley
Colors: Green, Orange, Silver, Red

1. Mr. Turner (who isn't Matt) finished one place ahead of the red club juggler.

2. The four are Leon, Mr. Vertigo, the one who juggled with orange clubs, and the one who came in third place.

3. Kyle finished behind (but not one place behind) Mr. Wheeley.

4. Matt and Mr. Pettle finished consecutively, in some order.

5. The one who juggled with green clubs finished two places behind Jake.

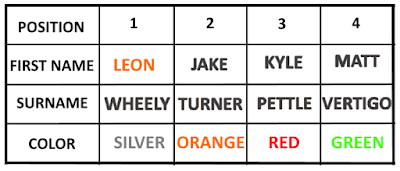
Know here final stats of the race! 

In The Race of Multi-Tasking Clowns

What was the puzzle?

Let's take a look at the given data.

------------------------------------------------------------

Places: 1st, 2nd, 3rd, 4th

First Names: Kyle, Matt, Jake, Leon

Surnames: Turner, Pettle, Vertigo, Wheeley

Colors: Green, Orange, Silver, Red


1. Mr. Turner (who isn't Matt) finished one place ahead of the red club juggler.

2. The four are Leon, Mr. Vertigo, the one who juggled with orange clubs, and the one who came in third place.

3. Kyle finished behind (but not one place behind) Mr. Wheeley.

4. Matt and Mr. Pettle finished consecutively, in some order.

5. The one who juggled with green clubs finished two places behind Jake.  

------------------------------------------------------------ 

We will make a table like below and fill it step by step.

 
 STEPS :

1] As per clue (2), Leon, Mr. Vertigo, the one who juggled with orange clubs, and the one who came in third place are supposed to be different. Hence, Leon or Vertigo didn't finish at 3. So, Pettle or Turner must be at 3.

2] But as per (1), Matt isn't a Turner, and as per (4) Matt and Pettle are two different persons. Hence, Matt didn't finish at 3 for sure.

3] As per (3), Mr. Wheely too can't be at 3 as in that case no placement would be left for Kyle. And Jake too can't be at 3 as in that case no placement would be left for the one who juggled with green clubs.

So, if Leon, Matt and Jake aren't at 3 then Kyle must be at 3 having surname Pettle or Turner.

4] With that, Mr. Wheely must have finished first as clue (3) suggests.

5] Now, as per (1), Turner didn't finish at no.4 since there would be no place for red club juggler. Also, as per (4), Pettle can't be at 4 since Matt can't be at 3 (STEP 3). Hence, at no.4, Vertigo is there.

6] As per (2), Vertigo and Leon are 2 different contestants. Also, as per clue (5) Jake can't be at no.4 as in that case there would be no place left for the one who juggled green clubs. Hence, the first name of Vertigo is Matt.

7] Now, clue (4) suggests Pettle is at no.3

8] The only place for the Turner is no.2 and by (1), Kyle Pettle is red club juggler.

9] Now, the only locations left for the contestants pointed by clue (5) i.e. for Jake and green club juggler are 2 and 4.

10] The only location left for Leon is no.1 and as per (2), Leon and orange club juggler are different. Hence, Leon must be a silver club juggler and Jake Turner is orange club juggler.

CONCLUSION : 

So, the final stats of race are - 

   

Who is the President of Logitopia?

Larry, Matt, and Nick live in the strange country of Logitopia. 

This country is inhabited by three races of people: the type A people who always tell the truth, the type B people who always lie, and the type C people who alternately tell the truth and lie. One of the three is the president of Logitopia.

Larry makes these two statements:

1. "The president is of a different race from the other two."
2. "Matt is not the president."

Matt makes these two statements:

1. "The president is a type B person."
2. "Larry is not the president."

Nick makes these two statements:

1. "Exactly two of us are of the same race."
2. "I am not the president."

Who is the president?

Know the NAME of the President!
 

Larry is the President of Logitopia

What was the puzzle?

First thing to note that it's not mentioned any where that three people are three different types; there may be 2 who are the same type.

Let's have a look at the statements made by 3.

Larry makes these two statements:

1. "The president is of a different race from the other two."
2. "Matt is not the president."

Matt makes these two statements:

1. "The president is a type B person."
2. "Larry is not the president."

Nick makes these two statements:

1. "Exactly two of us are of the same race."
2. "I am not the president."
  
We'll refer Larry's statements as L1 & L2, Matt's as M1 & M2 and those of Nick's as N1 & N2.

ANALYSIS : 

1] Suppose Matt's first statement M1 is TRUE. So, he is not a TYPE B person for sure & hence not the president. Therefore, L2 must be TRUE making sure Larry is not TYPE B person nor a president. So, Nick must be the president & TYPE B person whose both statements are FALSE. Since, N1 is turning out to be FALSE, neither Matt nor Larry can be TYPE B or both of them can't be of the same type i.e. TYPE A or TYPE C. 

However, since M2 turns out to be TRUE, Matt must be TYPE A person and hence leaving Larry as a TYPE C person. In that case, since L2 is TRUE, L1 must be FALSE. But in the case the president (TYPE B) is really different from the other two (TYPE A and TYPE C) making L1 TRUE. So, the assumption that M1 is TRUE goes wrong here in the case.

2] Now, let's suppose that Matt himself is the president. Then, L2 must be FALSE and M2, N2 would be TRUE. Since, M2 is TRUE, Matt (the president) can't be TYPE B & we know M1 is FALSE,  Matt must be TYPE C person & president.

Anyhow, Larry's first statement L1 can't be TRUE as in that case, he too will be TYPE C person as president Matt which is against the statement L1 itself. Since, his other statement L2 is FALSE, he must be TYPE B person.

Now, with N2 to be TRUE, if N1 is assumed to be TRUE then Nick would be TYPE A person. So, all three would belong to 3 different races which contradicts statement N1 itself. Hence, N1 must be FALSE. 

And if N1 FALSE and N2 TRUE, Nick would be TYPE C person as president Matt while Larry being TYPE B person. But this makes statement N1 TRUE which again contradicts our conclusion above. 

Therefore, Matt too can't be the president.

3] Suppose Nick is the president. That would make N2 FALSE and L2, M2 TRUE.
As concluded in STEP 1 above, we know M1 has to be FALSE. Then the President Nick can't be TYPE B person. With one of his false statement N2, Nick must be TYPE C person. Therefore, N1 must be TRUE. So, both Matt and Nick are TYPE C persons. 

That indicates president isn't of a different race than other two. That is L1 turns out to be FALSE. With L2 proved TRUE already, Larry would be TYPE C person. So all three would be TYPE C which contradicts TRUE statement N1.

4] Therefore, Larry must be the president.

That is L2, N2 must be TRUE and M2 must be FALSE. With M1 proved FALSE already in [1] above, Matt must be TYPE B person. Nick can't be TYPE B person with TRUE N2. 

If N1 is TRUE (i.e. Nick is TYPE A person) then Larry must be the other person with TYPE A (since Matt is TYPE B person) along with Nick for N1 to be TRUE. So, L1 too has to be TRUE. But, the president Larry is of the same race as that of Nick which is against L1 itself.

Hence, N1 must be FALSE and Nick must be TYPE C person. And therefore, L1 has to be TRUE making president Larry as a TYPE A person.

CONCLUSION : 

The President of Logitopia is Larry who is TYPE A person. Matt is TYPE B person and Nick is TYPE C person. 

Who Will Win the Race? You or I ?

Here’s a long corridor with a moving walkway. Let’s race to the far end and back. We’ll both run at the same speed, but you run on the floor and I’ll run on the walkway, going “downstream” to the far end and “upstream” back to this point. 

Who will win?

This person will be the winner of the race!

"You Will Be Winner of the Race!"

How race was conducted?

Let's assume that we have to run 60 units forward & 60 units backward i.e. total 120 units of distance.

Let 10 units be the speed of the moving walkway. Then I have to run faster than 10 while coming back "upstream" to reach at the source again.

So let 20 units be the our speed of running.

Speed = Distance/Time

Time = Distance/Speed

Time that you need to complete the race = 120/20 = 6 unit.

Time that I need to go forward = 60/(20+10) = 2 units.

Time that I need to come back = 60/(20-10) = 6 units.

Hence, 

Time that I need to complete the race = 4 + 12 = 8 units.

I will require more time to complete the race, that's why you will be the winner of the race!  

The Race Between 2 Brothers

Zachary challenges his brother Alexander to a 100-meter race. Alexander crosses the finish line when Zachary has covered only 97 meters.

The two agree to a second race, and this time Alexander starts 3 meters behind the starting line.

 
If both brothers run at the same speed as in the first race, who will win?

He will win the second race for sure! 

Source 

Winner of The Race Between 2 Brothers

What was the race of?

Let's assume that, Alexander completes the first race in time 't'. That means, he reaches at the finish line after running 100m after time 't' since start of the race. In the same time interval, Zachary could reach only 97m.

Now, in second race too, Alexander covers 100m once again in time interval 't' & Zachary runs 97m distance. Since, Alexander started 3m ahead of start line, at this point of time both are at the same point with 3m left to complete the race. 

 
Since, Alexander had won first race with faster speed & speed of both are unchanged in second race, it's clear that Alexander will take less time to cover leftover 3m distance. Hence, Alexander will be winner of the second race. 

MATHEMATICAL APPROACH:

Let's suppose Alexander took 10s to complete the first race. Then, his speed is 100/10 = 10m/s.

In 10s, the Zachary could run only 97m. So, his speed is 97/10 = 9.7m/s.

In the second race, their respective speeds are unchanged but Alexander has to run 103m to reach at the finish line compared to 100m of Zachary.

Hence, time taken by Alexander to reach at the finish line = 103/10 = 10.3s and that taken by Zachary = 100/9.7 = 10.30929s.

It's clear that Zachary needs more time to finish this race too. Hence, Alexander will be the winner in this race as well. He beats Zachary by 100 - (10.3x9.7) = 0.09m.

Cars Around Interesting Circular Track

Around a circular race track are n race cars, each at a different location. At a signal, each car chooses a direction and begins to drive at a constant speed that will take it around the course in 1 hour. When two cars meet, both reverse direction without loss of speed. Show that at some future moment all the cars will be at their original positions.

Here is that proof!


Source 

Analysing Interesting Circular Race Track

What was the interesting fact about?

Just imagine that each car carries a flag on it and on meeting pass on that flag to the next car. Obviously, this flag will move at the constant speed around the track as cars carrying it are also moving at the constant speed. So, the flag will be back at the original position after 1 hour.

Let's assume there are only 2 cars on the track at diagonally opposite points as shown below. 

After 15 minutes, on meeting with Car 2, Car 1 will pass on the flag & both will reverse their own direction. 30 minutes later (i.e. 45 minutes after start) both cars again meet each other and Car 2 will pass on flag back to Car 1 & directions are reversed again. Again in another 15 minutes (i.e. after 1 hour from start), both cars are back at the original positions. 

Now, let's suppose that there are 4 cars on the track positioned as below.

The above image shows how cars will be positioned after different points of time & how they reverse direction after meeting.

Again, all are back to the original position after 1 hour including the flag position. One more thing to note that the orders in which cars are never changes. It remains as 1-2-3-4 clockwise. 

To conclude, for 'n' number of cars, at some point of time all the cars will be in original positions in future.   
 

100m Running Race

Lavesh, Bolt, and Lewis race each other in a 100 meters race. All of them run at a constant speed throughout the race.

Lavesh beats Bolt by 20 meters.
Bolt beats Lewis by 20 meters.

How many meters does Lavesh beat Lewis by ? 

Know here the answer! 

Source 

Winner Beats Second Runner Up by...

What was the question? 

Let Lavesh's speed be 10 m/s. Then he must have taken 10 seconds to finish the race. Since Bolt was beaten by Lavesh by 20 m he must have run 80m when Lavesh finished race in 10 seconds (t=10). So his speed would be 8 m/s.

Now Bolt requires 100/0.8 = 12.5 seconds to finish the race. When he finished, Lewis was 20m behind i.e. 80m from starting point at t = 12.5. So Lewis speed is 80/12.5 = 6.4 m/s

At t = 10 seconds, when Lavesh finished his race Lewis must be at 6.4 x 10 = 64 m from starting point. Hence Lavesh beats Lewis by 100 - 64 = 36 m.

Another method.

Let L be the speed of Lavesh, B be the speed of Bolt & W be that of Lewis. Then,

L/B = 100/80 = 5/4

L = (5/4) B  .......(1)

Similarly,

B/W = 100/80 = 5/4

B = (5/4) W .......(2)

Putting (2) into (1),

L = (5/4) x (5/4) W

L/W = 25/16

L = (25/16) W

For given time t, when Lavesh finished the race,

Distance by L/ Distance by W = 25 / 16

100 m/ Distance by W = 25 / 16

Distance by W = (16 x 100) / 25 = 64.

  
So when Lavesh finished cross line at 100 m, Lewis was at 64m i.e. 36m behind. In other words, Lavesh beats Lewis by 100 - 64 = 36 m.

Finding Horses For Courses

There are 25 horses among which you need to find out the fastest 3 horses. You can conduct race among at most 5 to find out their relative speed. At no point you can find out the actual speed of the horse in a race. Find out how many races are required to get the top 3 horses. 

Click here for logical answer! 

Source 

Races To Find Horses For Courses

What was the task? 

First we need to make 5 groups of 5 horses each. Let's put each group on race & note down rank of each horse & corresponding group. This requires 5 races.

Taking out winner of each group aside. In a next race, winner of each group run & fastest winner is found. Total 6 races are conducted till now.

Now let's name group of fastest winner as A, second fastest winner as B & so on as C, D, E. Each horse would be identified by it's group name & rank as Group.Rank. For example, B.3 means the horse that came 3rd in group B & D.5 is horse that came last in group D.

For next race, we can eliminate few. Let's remind we have to find 3 fastest horses. Now let's logically eliminate few horses. Those horse for which we are sure that at least other 3 are faster than those will be eliminated.

1. Now A.1, B.1 & C.1 are faster than D.1 & E.1. So D.1 & E.1 & respective groups eliminated straightaway.

2. The C.2 (& other members of C) eliminated as A.1, B.1, C.1 are faster ones.

3. The B.3 (& other member of B) eliminated as at least A.1, B.1, B.2 are faster ones.

4. Similarly, A.4 & A.5 have 3 horses ahead as A.1, A.2 & A.3.

5. A.1 is already proved it's fastest among all, so no need to re race by taking it.

Complex Time, Speed & Distance Maths

There is a circular race-track of diameter 1 km. Two cars A and B are standing on the track diametrically opposite to each other. They are both facing in the clockwise direction. At t=0, both cars start moving at a constant acceleration of 0.1 m/s/s (initial velocity zero). Since both of them are moving at same speed and acceleration and clockwise direction, they will always remain diametrically opposite to each other throughout their motion.

At the center of the race-track there is a bug. At t=0, the bug starts to fly towards car A. When it reaches car A, it turn around and starts moving towards car B. When it reaches B, it again turns back and starts moving towards car A. It keeps repeating the entire cycle. The speed of the bug is 1 m/s throughout.

After 1 hour, all 3 bodies stop moving. What is the total distance traveled by the bug?

Find out complex calculations here!

Simple Solution of Complex Problem

Here is that complex looking problem! 

Everything built, written or designed in the given problem is to distract you from basic physics formula.

Speed = Distance / Time

Hence,

Distance = Speed x Time 

All the details given except speed of bug & time for which it traveled are there to confuse you. Speed of bug is 1 m/s & it traveled for 1 hour = 3600 seconds.

Distance = 1 x 3600 = 3600 m

So the total distance traveled by the bug is 3600 m.