Skip to main content
Posts
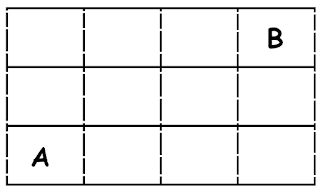
Consider a rectangular grid of 4×3 with lower left corner named as A
and upper right corner named B. Suppose that starting point is A and you
can move one step up(U) or one step right(R) only. This is continued
until B is reached.
How many different paths from A to B possible ?
Here is calculation of total number of paths.
What is the question?
If the right move is represented as R and up move as U then, RRRUU is the one path to reach at B.
UURRR is one more path between points A and B.
URRRU is another way to reach at B.
Further, one can reach at B via RURRU.
So number of such paths are possible.
However, if all paths above are observed, we can conclude that total 5 moves are needed to reach from point A to B. Out of those 5, 3 have to be RIGHT and 2 have to be UP.
That is, any combination having 3R and 2U in 5 moves will give a valid path to reach at B.
Now, number of ways 3R can be placed in 5 moves can be calculated as -
C(5,3) = 5!/(5-3)! * 2! = 10.
To sum up, there are 10 paths available between points A and B.