Skip to main content
Posts
Can you break this code?
Shift three. Caps lock one. Shift six.
Tab six. Tab nine. Tab seven.
Tab four. Tab three. Caps lock one. Caps lock three.
Tab five. Caps lock six. Tab eight. Caps lock two. Shift 10.
Know here what that code is!
Title is not the question; question is here!
The given code is -
Shift three. Caps lock one. Shift six.
Tab six. Tab nine. Tab seven.
Tab four. Tab three. Caps lock one. Caps lock three.
Tab five. Caps lock six. Tab eight. Caps lock two. Shift 10.
If carefully observed the code, it represents keyboard like a grid/table.
Shift, Caps lock, Tab represents rows while the numbers associated with them represents a column. Together both represents a particular key on the keyboard.
Shift three - C Caps lock one - A Shift six - N
Tab six - Y Tab nine - O Tab seven - U
Tab four - R Tab three - E Caps lock one - A Caps lock three - D
Tab five - T Caps lock six - H Tab eight - I Caps lock two - S
Shift 10 - ?
So the code is CAN YOU READ THIS?
There is a ten-digit mystery number (no leading 0), represented by
ABCDEFGHIJ, where each numeral, 0 through 9, is used once.
Given the
following clues, what is the number?
1) Digit A is either a square number or a triangle number, but not both.
2) Digit B is either an even number or a cube number, but not both.
3) Digit C is either a cube number or a triangle number, but not both.
4) Digit D is either an odd number or a square number, but not both.
5) Digit E is either an odd number or a cube number, but not both.
6) Digit F is either an odd number or a triangle number, but not both.
7) Digit G is either an odd number or a prime number, but not both.
8) Digit H is either an even number or a square number, but not both.
9) Digit I is either a square number or a cube number, but not both.
10) Digit J is either a prime number or a triangle number, but not both.
11) A < B, C < D, E < F, G < H, I < J
12) A + B + C + D + E < F + G + H + I + J
Here is that MYSTERY number!
The FIRST Case of Mystery Number
The SECOND Case of Mystery Number
What was the challenge?
Taking a look at the given clues first for identifying the mystery number ABCDEFGHIJ.
---------------------------------------------------------------------------------
1) Digit A is either a square number or a triangle number, but not both.
2) Digit B is either an even number or a cube number, but not both.
3) Digit C is either a cube number or a triangle number, but not both.
4) Digit D is either an odd number or a square number, but not both.
5) Digit E is either an odd number or a cube number, but not both.
6) Digit F is either an odd number or a triangle number, but not both.
7) Digit G is either an odd number or a prime number, but not both.
8) Digit H is either an even number or a square number, but not both.
9) Digit I is either a square number or a cube number, but not both.
10) Digit J is either a prime number or a triangle number, but not both.
11) A < B, C < D, E < F, G < H, I < J
12) A + B + C + D + E < F + G + H + I + J
---------------------------------------------------------------------------------
STEPS :
Even numbers between 0 to 9 - 0, 2, 4, 6, 8
Odd numbers between 0 to 9 - 1, 3, 5, 7, 9
Square numbers between 0 to 9 - 0, 1, 4, 9
Cube numbers between 0 to 9 - 8.
Prime numbers between 0 to 9 - 2, 3, 5, 7
Triangle numbers between 0 to 9 - 0, 1, 3, 6
---------------------------------------------------------------------------------
STEP 1 :
A can't be 0 as no leading 0's in the mystery number ABCDEFGHIJ.
Remember both of hints can't be true at the same time. That is Digit A is either a square or a triangle number but not both. Since, 0 and 1 are square as well as triangle numbers, A can't be 0 or 1.
Similarly, 8 is even as well as cube, B can't take 8. And so on.
Possible values of A - 3, 4, 6, 9. (Square/Triangle)
Possible Values of B - 0, 2, 4, 6. (Even/Cube).
Possible Values of C - 0, 3, 6, 8. (Cube/Triangle)
Possible Values of D - 3, 4, 5, 7. (Odd/Square)
Possible Values of E - 3, 5, 7, 8. (Odd/Cube)
Possible Values of F - 0, 5, 6, 7, 9 (Odd/Triangle)
Possible Values of G - 1, 2, 9 (Odd/Prime)
Possible Values of H - 0, 2, 6, 8, 9 (Even/Square)
Possible Values of I - 4, 8, 9 (Square/Cube)
Possible Values of J - 0, 1, 2, 5, 6, 7 (Prime/Triangle)
---------------------------------------------------------------------------------
STEP 2 :
The value of I can't be 8 or 9 as there will be no digit left for J which is greater than I.
Hence, I = 4.
---------------------------------------------------------------------------------
STEP 3 :
Digit A can't be 6 or 9 since no digit will be left for B>A.
As we have, I = 4, the digit A = 3 and hence B = 6.
---------------------------------------------------------------------------------
STEP 4 :
The only digit left for C is 0, i.e. C = 0 as C can't be 8 for C<D to be true.
---------------------------------------------------------------------------------
STEP 5 :
Since I = 4 itself, so digit J can't be 0, 1, 2 for I<J to be true.
Digit D and digit J take either take 5 or 7. With that the only valid digit left for E is 8 to satisfy the condition E<F.
Hence E = 8.
---------------------------------------------------------------------------------
STEP 6 :
If E = 8, then F has to be 9. So, F = 9.
---------------------------------------------------------------------------------
STEP 7 :
With 6, 8, 9 already taken up by other letters, the only digit left for H is 2 for G<H to be true. So, H = 3 and hence G = 1.
---------------------------------------------------------------------------------
STEP 8 :
As per condition 12,
A + B + C + D + E < F + G + H + I + J
3 + 6 + 0 + D + 8 < 9 + 1 + 2 + 4 + J
17 + D < 16 + J
As deduced in STEP 4, D must be 5 or 7 and J must be 7 or 5.
For above equation to be true, D = 5 and J = 7.
---------------------------------------------------------------------------------
Conclusion :
A = 3, B = 6, C = 0, D = 5, E = 8, F = 9, G = 1, H = 2, I = 4, J = 7
Hence, the number ABCDEFGHIJ must be 3605891247.
Verifying the given hints -
1) 3 is a triangle number, but not a square number.
2) 6 is an even number, but not a cube number.
3) 0 is a cube number, but not a triangle number.
4) 5 is an odd number, but not a square number.
5) 8 is a cube number, but not an odd number.
6) 9 is an odd number, but not a triangle number.
7) 1 is an odd number, but not a prime number.
8) 2 is an even number, but not a square number.
9) 4 is a square number, but not a cube number.
10) 7 is a prime number, but not a triangle number.
11) 3 < 6, 0 < 5, 8 < 9, 1 < 2, 4 < 7
12) 3 + 6 + 0 + 5 + 8 = 22, 9 + 1 + 2 + 4 + 7 = 23, 22 < 23
The Antisocial Club meets every week at Jim's Bar. Since they are so
antisocial, however, everyone always sits as far as possible from the
other members, and no one ever sits right next to another member.
Because of this, the 25-stool bar is almost always less than half full
and unfortunately for Jim the members that don't sit at the bar don't
order any drinks.
Jim, however, is pretty smart and makes up a new
rule: The first person to sit at the bar has to sit at one of two
particular stools. If this happens, then the maximum number of members
will sit at the bar.
Which stools must be chosen? Assume the stools
are numbered 1 to 25 and are arranged in a straight line.
Wise Selection of first 2 Seats made available!
What was the challenge?
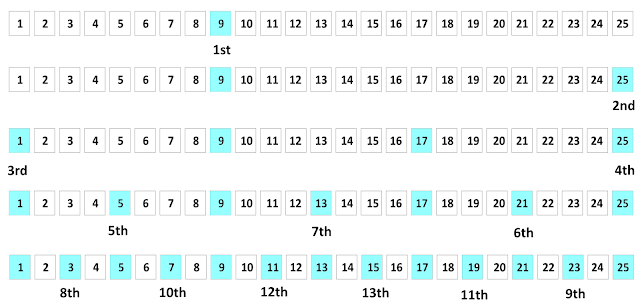
The bar owner Jim put only 2 options for first person to seat and those options are Stool No. 9 and Stool No.17. We'll call seat for stool for convenience.
Choice of numbers 9 and 17 is because of symmetry explained below.
Suppose the first person choose seat no.9.
Then the second person has to choose the furthest seat i.e. seat no.25.
The third person will choose seat no.1 which is furthest from seat no.9 and 25.
The fourth person won't have option other than seat no.25 which is the furthest from rest of 3.
For the fifth person, seats numbered 5, 13 and 21 are available which are equidistant from 2 persons. We assume he chooses farthest from the seat occupied by person who entered just ahead of him. That is he chooses seat no.5.
Since, seat no.21 is furthest from seat no.5 and only seat equidistant from 17 & 25, the sixth person will choose seat no.21.
With that, 7th person won't have any option other than seat no.13 to maintain distance from others.
With the same logic, next 6 persons occupies seat numbered 3, 23, 7, 19, 11, 15.
So there will be 13 people maximum in the bar with no one seating next to each other.
The same seat numbers will be occupied even if the first person choose seat no.17. The second person will choose seat no.1, third will choose seat no.25, fourth will choose seat no.9 and so on.
But if the first person doesn't choose 9 or 17 then there can be less than or equal to 12 people maximum in the bar following their antisocial trait.
There is a ten-digit mystery number (no leading 0), represented by
ABCDEFGHIJ, where each numeral, 0 through 9, is used once. Given the
following clues, what is the number?
1) Either A = B / 3 or A = G + 3.
2) Either B = I - 4 or B = E + 4.
3) Either C = J + 2 or C = F * 3.
4) Either D = G * 4 or D = E / 3.
5) Either E = J - 1 or E = D / 4.
6) Either F = B * 2 or F = A - 4.
7) Either G = F + 1 or G = I - 3.
8) Either H = A / 2 or H = C * 3.
9) Either I = H + 3 or I = D / 2.
10) Either J = H - 2 or J = C * 2.
HERE is that MYSTERY Number!
What was the FIRST case?