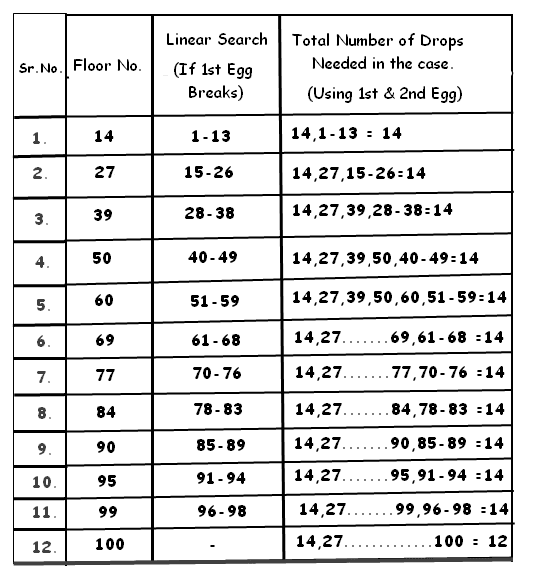
"Get Out of The Hell !"

You’re new to hell, and you’re given a choice: You can go directly to the fourth circle, or you can play simultaneous chess games against Alexander Alekhine and Aron Nimzowitsch. Alekhine always plays black and smokes a pipe of brimstone. Nimzowitsch plays white and wears cuff links made of human teeth. Neither has ever lost.

If you can manage even a draw against either player, you’ll be set free. But if they both beat you, you’ll go to the eighth circle for eternity.

What should you do?

This trick will save your life! 

Freedom From The Hell !

How you are trapped in hell?

Alekhine always plays black and Nimzowitsch plays always white. Obviously, you will be forced to choose white against Alekhine and black against Nimzowitsch. 

Wait for Nimzowitsch's first move and then play the same move on Alekhine’s board. Note how Alekhine responds to move & copy that move on Nimzowitsch's board.

This way, effectively Nimzowitsch is playing against Alekhine and you are just transferring moves between to 2 masters.

Since, they never lost a single chess game, there are high chances that the game between two ends in draw.

Even if Alekhine wins with his black then you are winning against Nimzowitsch as you are copying Alekhine's move against Nimzowitsch's white using your black. Same is case if Nimzowitsch wins.

In short, you will end up with either draw with both or win against at least one (against both is impossible). You will be free in any case.

And in fact, you need not to have knowledge of how to play chess to get freedom from the hell.

Probability of The Correct Answer?

This is a popular probability puzzle in which you have to select the correct answer at random from the four options below.

Can you tell, whats the probability of choosing correct answer in this random manner.

1) 1/4
2) 1/2
3) 1
4) 1/4

And the correct answer is......

Finding The Probability of Correct Answer

What was the question?

It can't be 1/4 as 1/4 appears 2 times in given 4 options as probability of correct answer when random selection of option in that case would be 2/4 = 1/2. This will be contradiction.

It can't be 1/2 either since the probability of the 1 correct answer out of 4 available options on random selection would be 1/4. That will be contradiction again.

It can't be 1 too as again probability of the 1 correct answer out of 4 available options on random selection would be 1/4. Once again this is contradiction.

Hence, the probability of the 1 correct answer out of 4 available options on random selection would be 0.

Dropping Height For Egg Breakdown!

There is a building of 100 floors.

-If an egg drops from the Nth floor or above it will break.

-If it’s dropped from any floor below, it will not break.

You’re given 2 eggs.

Find N.

How many drops you need to make?

What strategy should you adopt to minimize the number egg drops it takes to find the solution?

'This' should be the strategy! 

Finding The Dropping Height For Egg Breakdown

What was the task?

Let's suppose, the we drop the egg from N the floor & it breaks then we need to do linear search till (N-1) th floor. For example, we dropped egg from 10 the floor & it breaks then we need to test 1-9 floors using 2nd egg to find the exact floor from which the egg breaks on dropping. So the best case would be 10 drops if the first egg breaks when dropped from 10 the floor.But that's only possible if the egg breaks on drop from 10th floor.

The worst case would be when egg doesn't break at 10,20,30,40,50,60,70,80,90 and breaks at 100th floor. Here, once again 91-99 need to be tested using other egg to find exact floor from which egg will break on drop.So in worst case, 19 drops would be needed.

Best way to minimize the number of drops required is to minimize the linear search (91-99 above  in worst case) that we need to do with the second egg after 1st egg breaks on drop from particular floor (100th floor in above worst case).

So after dropping egg from N th floor & if egg doesn't break then instead of going to next Nth floor, better to go N + (N-1) th floor. And now if the egg break here at N + (N-1) th floor then we need to do linear search from (N+1) th floor to N + (N-1) th floor instead of (N+1) th floor to (N + N) th floor. That's 1 less linear search than that needed if we go to the next N th floor if egg doesn't break on drop from Nth floor.

After dropping egg from N + (N-1) th floor, if it doesn't break then we should go the the N + (N-1) + (N-2) th floor.

Adding all instances of drops i.e. drop at Nth, drop at N + (N-1) th , drop at N + (N-1) + (N-2) & so on gives us

N + (N-1) + (N-2) + (N-3).......+1 = N(N+1)/2.

which shouldn't exceed 100 as there are only 100 floors & hence total number of drops must not be greater than 100.

So,

N(N+1)/2 >= 100

N^2 + N - 200 >= 0

This is

This is quadratic equation in form ax^2 + bx + c = 0 where x = [-b +- (b^2 -4ac)^0.5]/2a.

Solving above for N gives,

N = 13.651

Rounding value of N to 14 in the case.

So we should start with floor no. 14 followed by 27,39,50,60,69,77,84,90,95,99,100

In the worst case here total drops needed are only 14. 

For example, if the egg breaks when dropped from 14th floor then for second egg we need to test 1-13 floors to find the exact floor from which egg breaks on the drop. See table below.

Similarly, for example, egg breaks after drop from 50 floor (after testing 14,27,39), we need to test at 51-59 floors by dropping second egg to know the exact floor from where egg breaks on the drop.
 

Find Numbers For The Boxes

Find the correct number to fill in the boxes below.

These should be correct numbers!

Finding Correct Numbers For The Boxes

How boxes are placed?

Let's assume a, b, c and d are the correct numbers in the boxes.

So, 4 equations that we get are,

a + b = 8     .....(1)

c - d = 6     .....(2)

a + c = 13   .....(3)

b + d = 8    .....(4)

Subtracting (1) from (3) gives,

c - b =  5     .....(5)

Adding (2) to (4),

c + b = 14    .....(6)

Adding (5) and (6) gives,

2c = 19

c = 9.5

Putting c = 9.5 in (2),

9.5 - d = 6

Hence, d = 3.5

Putting d =3.5 in (4),

b + 3.5 = 8

b = 4.5

Putting b = 4.5 in (1),

a + 4.5 = 8

a = 3.5

So to conclude, a = 3.5, b = 4.5, c = 9.5 and d = 3.5.

The Royal Love Story!

You’re a knight in love with a princess. Unfortunately, the king knows you’re poor and disapproves of the match.

On the night of a great feast, the king calls you up before his men and presents a golden box. In it are two folded slips of paper. One, he announces, reads “Marriage,” the other “Death.” “Choose one,” he says.

Pretending to stir the fire, the princess manages to whisper that both slips say “Death.” But the king and his men are waiting, and you cannot escape now.

What should you do?

This is how you can escape! 

Getting Your Love - 'The Princess'

What was the biggest challenge in getting that?

All you need to do is take either slip & drop it into the fire.

Now you can say, "Sorry, I couldn't manage to read my fate. But we have option to read what it was. It must be the opposite of what other slip reads.".

And obviously, other slip has 'Death' is written so no one can object that one that dropped in fire must had 'Marriage'. Any claim saying other too had 'Death' would raise the question of credibility of the king directly; so no one would dare to claim that.

The Apple Conundrum

Two women are selling apples. The first sells 30 apples at 2 for $1, earning $15. The second sells 30 apples at 3 for $1, earning $10. So between them they’ve sold 60 apples for $25.

The next day they set the same goal but work together. They sell 60 apples at 5 for $2, but they’re puzzled to find that they’ve made only $24.

What became of the other dollar?

Here, could be that lost dollar! 

Behind The Apple Conundrum

What is the conundrum?

They sell 60 apples at 5 for $2, that means 12 such sets of 5 apples. Suppose, out of each such set, 1 woman takes out $1 for 2 apples and other takes $1 for 3 apples. So, first woman earns $12 by selling 24 apples and second woman sells 36 apples for $12.

In short, first woman gives away 6 apples (from her 30 apples) to second woman increasing her count to 36 reducing her own count to 24. First woman would have made $3 from those but second woman only made $2 from those 6 apples. And there is that lost dollar in earning.

So, 60 apples can't be divided equally to find the earning as they had sold apples at different rates on previous day.

Other way, if they wanted to sell apples together with 30 apples each, then they should have sold apples at average of (1/2 + 1/3)/2  = $5/12 per apple (i.e. 12 apples for $5) instead of $2/5 per apple.

The difference in price per apple (5/12 - 2/5) = (1/60).

So the difference in earning after selling 60 such apples = (1/60) x 60 = 1.

And there is that other dollar! 

What's wrong gone here on next day? Instead of averaging dollars per apple, apples per dollar are added directly which resulted reduced cost of each apple. 

The Mistimed Clock!

Andrea’s only timepiece is a clock that’s fixed to the wall. One day she forgets to wind it and it stops.

She travels across town to have dinner with a friend whose own clock is always correct. When she returns home, she makes a simple calculation and sets her own clock accurately.

 
How does she manage this without knowing the travel time between her house and her friend’s?


That's how she manages to set it accurately!
 

Correcting The Mistimed Clock!

 How it was mistimed?

Andrea winds her clock & sets it to the arbitrary time. Then, she leaves her house and when she reaches her friend's house, she note down the correct time accurately. Now, after having dinner, she notes down the correct time once again before leaving her friend's house.

After returning to home, she finds her own clock acted as 'timer' for her entire trip. It has counted time that she needed to reach her friend's house + time that she spent at her friend's house + time she needed to return back to home.

Since, Andrea had noted timings at which she reached & left her friend's house, she can calculate the time she spent at her friend's house. After subtracting this time duration from her unique timer count she gets the time she needed to reach to & return from her friend's house.

She must have taken the same time to travel from her home to her friend's home and her friend's home to her home. So dividing the count after subtracting 'stay time' she can get how much time she needed to return back to home.

Since, she had noted correct time when she left her friend's home, now by adding time that she needed to return back to home to that, she sets her own clock accurately with correct time.

Let's try to understand it with example.

Suppose she sets her own clock at 12:00 o' clock and leave her house. Suppose she reaches her friend's home and note down the correct time as 3:00 PM. After having dinner she leaves friend's home at 4:00 PM.

After returning back to home she finds her own clock showing say 2:00 PM. That means, she spent 120 minutes outside her home with includes time of travel to and from friends home along with time for which she spent with her friend. If time of stay at her friend is subtracted from above count, then it's clear that she needed 60 minutes to travel to & return back from friend's home.

That is, she needed 30 minutes for travel the distance between 2 homes. Since, she had noted correct time as 3:00 PM when she left friend's home, she can set her own clock accurately at 3:30 PM.

Tricky Probability Puzzle of 4 Balls

I place four balls in a hat: a blue one, a white one, and two red ones. Now I draw two balls, look at them, and announce that at least one of them is red. What is the chance that the other is red?

Well, it's not 1/3!

Tricky Probability Puzzle of 4 Balls : Solution

What was the puzzle?

It's not 1/3. It would have been 1/3 if I had taken first ball out, announced it as red and then taken second ball out. But I have taken pair of ball out. So, there are 6 possible combinations.

red 1 - red 2
red 1 - white
red 2 - white
red 1 - blue
red 2 - blue
white - blue 

Out of those 6, last is invalid as I already announced the first ball is red. That leaves only 5 valid combinations.

And out of 5 possible combinations only first has desired outcome i.e. both are red balls.
Hence, there is 1/5 the chance that the other is red 

"Save Your House From Me!"

Okay, I’ll ask three questions, and if you miss one I get your house. Fair enough? Here we go:

1.A clock strikes six in 5 seconds. How long does it take to strike twelve?

2.A bottle and its cork together cost $1.10. The bottle costs a dollar more than the cork. How much does the bottle cost?

3.A train leaves New York for Chicago at 90 mph. At the same time, a bus leaves Chicago for New York at 50 mph. Which is farther from New York when they meet?

You need little common sense in answering above!

Presence of Common Sense in Answers!

What where questions?

1. A clock strikes six in 5 seconds. How long does it take to strike twelve?

A: Not 10 seconds, it takes 11 seconds.

Here, interval between 2 strikes is 1 second i.e. if counter started at first strike, it will count 1 second after second strike, 2 seconds after third strike & so on. 

Hence, 11 seconds needed for strike 12.

2.A bottle and its cork together cost $1.10. The bottle costs a dollar more than the cork. How much does the bottle cost?

A: Not 1 dollar, it would cost $1.05.

If x is cost of cork,
x + (x +1) = 1.10
2x = 0.10
x = 0.05

Hence cost of bottle is $1.05 and cost of cork is $0.05



3.A train leaves New York for Chicago at 90 mph. At the same time, a bus leaves Chicago for New York at 50 mph. Which is farther from New York when they meet?

A: Obviously, when they meet at some point then that point must be at the some distance from New York. Hence, they are at the same distance from the city.

40 Matchsticks Challenge!

The diagram below shows 40 matchsticks arranged in a square grid.

What is the fewest number of matchsticks that need to be removed so that there are no squares (of any size) remaining?


This is how it can be done! 

Eliminating Square in 40 Matchsticks Challenge!

What was the challenge?

All that we need to do is that remove these differently colored 9 matchsticks.

So we get, 

There can be other variations of this answer but we have to remove 9 matchsticks at least to make sure there is no square.

Who Will Win the Race? You or I ?

Here’s a long corridor with a moving walkway. Let’s race to the far end and back. We’ll both run at the same speed, but you run on the floor and I’ll run on the walkway, going “downstream” to the far end and “upstream” back to this point. 

Who will win?

This person will be the winner of the race!

"You Will Be Winner of the Race!"

How race was conducted?

Let's assume that we have to run 60 units forward & 60 units backward i.e. total 120 units of distance.

Let 10 units be the speed of the moving walkway. Then I have to run faster than 10 while coming back "upstream" to reach at the source again.

So let 20 units be the our speed of running.

Speed = Distance/Time

Time = Distance/Speed

Time that you need to complete the race = 120/20 = 6 unit.

Time that I need to go forward = 60/(20+10) = 2 units.

Time that I need to come back = 60/(20-10) = 6 units.

Hence, 

Time that I need to complete the race = 4 + 12 = 8 units.

I will require more time to complete the race, that's why you will be the winner of the race!