Puzzle : And Escape Story of Robbers Continues

Where story begins?

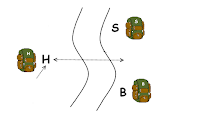
Babylas, Hilary, and Sosthenes have escaped the tower and divided their treasure into three bags. But now they must cross a river, and the boat can accommodate only two men at a time, or one man and a bag. None will trust another with his bag on the shore, but they agree that a man in the boat can be trusted to drop or retrieve a bag at either shore, as he’ll be too busy to tamper with it.

 How can they cross the river?

Source. 


This is how they can cross the river! 

 

Solution: Robbers' Planned Journey Across the River

Why they need a PLAN?

Let's recall that the boat can accommodate only two men at a time, or one man and a bag.

1. Sosthenes takes his bag across the river leaves it at other shore & comes back.

2. Sosthenes takes Hilari's bag to the other shore & leaves it there where his own bag is already there. 

3. Now, Hilari takes Sosthenes to the other shore, leaves him there & come back after recollecting own bag.

4. Hilari drops own bag at near shore & takes Babylas to other shore & returns back.

5. Next, he takes Babylas's bag & drops it at other shore where Babylas is waiting for his bag. And Hilary returns once again.

6. Finally, he collects his own bag and takes it to other shore.

The River Crossing Challenge!

There are 3 men, two Chimps, and one Gorilla on one side of a river :

• They have a boat but only the men and the Gorilla can row the boat across, so there must always be a human and/or Gorilla on the boat.

• The boat can only carry two people/monkeys.

• If monkeys and humans are together on one side of the river there must be as many or more people than monkeys for the men's safety. 

How can all men and monkeys make it to the other side ? 



Here is the PROCESS by which it can be done! 

Responding to The River Crossing Challenge!

What was the challenge ahead?

Recalling the conditions those need to be followed. 

--------------------------------------------------------------

• They have a boat but only the men and the Gorilla can row the boat across, so there must always be a human and/or Gorilla on the boat.

• The boat can only carry two people/monkeys.

• If monkeys and humans are together on one side of the river there must be as many or more people than monkeys for the men's safety.

--------------------------------------------------------------
 
Here, we go step by step process. (M - Men, G - Gorilla, C - Chimps)

1. The gorilla takes 1 chimp across the river and comes back. 

    (M - 3, G - 1, C - 1 | M - 0, G - 0, C - 1) 

2. Again, gorilla takes 1 man across the river and comes back. 

    (M - 2, G - 1, C - 1 | M - 1, G - 0, C - 1)

Now, here gorilla can't take chimp across the river as that will violate condition 3 on that side. Neither gorilla can take 1 man on other side and return back since number of monkeys on returning side will be more than people again violating condition 3.

3. Next, one man drops gorilla at the other side and bring back chimp.

    (M - 2, G - 0, C - 2 | M - 1, G - 1, C - 0) 

4. Now, 2 men has to cross the river and send back gorilla for the rest of work.

   (M - 0, G - 1, C - 2 | M - 3, G - 0, C - 0) 

5. Finally, gorilla takes 2 chimps across the river in 2 round trips.

   (M - 0, G - 0, C - 0 | M - 3, G - 1, C - 2) 

 

"How Did They Cross The River?"

Five men and five dogs (each man owned a dog) went hiking. They encountered a river that was swift and deep. The only way to cross it was an abandoned boat, left ashore on their side. But it would only hold three living things. 

Unfortunately, the dogs were edgy and could not be near another person (not even momentarily) unless its owner was present. One of the dogs attended a highly advanced, highly specialized obedience school and therefore knew how to operate the boat (as the men did) -- the other dogs lack this skill. 

How did the five men and the five dogs cross the river?

THIS is how they accepted the challenge! 

The Challenge of River Crossing

What was the challenge?

Let's name five men as M1, M2, M3, M4, M5 & five dogs as D1, D2, D3, D4, D5.
Assume D1 be the dog having advanced skills of operating boat.

TRAVEL CHART :

1] The dog D1 rows D2, D3 across the river and returns back. After returning back D1 takes D4 across the river & returns back once again.

START : D1-M1, D5-M5, M2, M3, M4  DESTINATION : D2, D3, D4

2] Now, M2, M3 and M4 cross river.

START : D1-M1, D1-M5  DESTINATION : D2-M2, D3-M3, D4-M4

3] Someone from DESTINATION needs to return back to allow others at START to cross the river. So, D4-M4 returns and D1-M1 cross the river. 

START : D4-M4, D5-M5  DESTINATION : D1-M1, D2-M2, D3-M3

4] After D1-M1 reaches to the DESTINATION, D3-M3 returns back.

START : D3-M3, D4-M4, D5-M5  DESTINATION : D1-M1, D2-M2

5] Now, M3, M4 and M5 cross the river.

START : D3, D4, D5  DESTINATION : D1-M1, D2-M2, M3, M4, M5.

6] Finally, the dog D1 at the DESTINATION returns back and makes 2 trips to take D3, D4 and D5 across the river.

START : None  DESTINATION : D1-M1, D2-M2, D3-M3, D4-M4, D5-M5.

This way, five men and five dog cross the river successfully.

Earnings Of People

Twenty men, women and children earn twenty coins between them. Each man earns 3 coins, each woman 1.5 coins and each child 0.5 coin.

How many men, women and children are there?

Find number of each of them here!

Number Of Earning People

What was the question? 

Let's suppose there are m men, w women and c children.

As per given data,

m + w + c = 20   ......(1)

3m + 1.5w + 0.5c = 20   .....(2)

Multiply equation (1) by 3 and then subtracting from (2), we get,

5m + 2w = 20.

For m and w to be whole numbers, m must be 2 and w must be 5 satisfying above equation.

Hence, from (1),

c = 20 - 2 - 5 = 13.

 
To conclude, there are 2 men, 5 women and 13 children.

Wise Men In Survival Game

A stark raving mad king tells his 100 wisest men he is about to line them up and that he will place either a red or blue hat on each of their heads.

Once lined up, they must not communicate among themselves. Nor may they attempt to look behind them or remove their own hat.The king tells the wise men that they will be able to see all the hats in front of them. They will not be able to see the color of their own hat or the hats behind them, although they will be able to hear the answers from all those behind them.

The king will then start with the wise man in the back and ask "what color is your hat?" The wise man will only be allowed to answer "red" or "blue," nothing more. If the answer is incorrect then the wise man will be silently killed. If the answer is correct then the wise man may live but must remain absolutely silent.The king will then move on to the next wise man and repeat the question.
 
The king makes it clear that if anyone breaks the rules then all the wise men will die, then allows the wise men to consult before lining them up. The king listens in while the wise men consult each other to make sure they don't devise a plan to cheat. To communicate anything more than their guess of red or blue by coughing or shuffling would be breaking the rules.

What is the maximum number of men they can be guaranteed to save?

Almost all can survive! Click here to know! 

Source 

Master Plan By Wise Men

Why this master plan needed? 

99 can be guaranteed to save! How?

Even if the person behind calls out the color of the hat that next person is wearing both would be survived only if they are wearing same color of hat. 

So how 99 can be saved?

For a simplicity, let's assume there are only 10 wise men & (only) assume we are among them. Now, we need to make a master plan to survive from this game of death.

One of us need to agree to sacrifice his life to save 9 of us & this person would be the first one in line. He will be survived of he has good luck.

The first person in line should shout RED if he founds number of RED hats even otherwise he should shout BLUE. Now if he has good luck then the hat color of his own hat would match & he would be survived.

The clue given by the first person is very important. Right from second person everyone need to count number of RED hats in front of him. Additionally, the next person need to keep track of number of RED hats that people behind him are wearing.