Sara's Desert Trek

Sara needs to trek from an oasis to a destination 10 miles away across a barren desert. 

The facts:

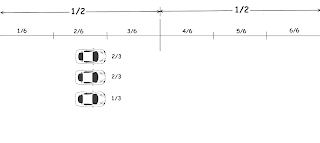
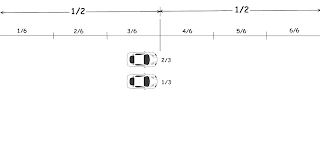
• Crossing one mile of desert requires using 1 gallon of water.
• Sara can only carry 6 gallons of water at a time.
• Sara can drop a water cache (of any amount of water from the supply she is carrying at that moment) at any of the nine stops along the route, and then pick up any part of the cache on a later trip.

What's the minimum number of times Sara must leave the oasis in order to cross the entire 10 mile span of desert?

This is how she optimizes her journey! 

Sara's Planning in Desert Trek

What was the challenge in journey?

1. First Sara collects 12 gallons of water at milepost 1 after having 3 trips from source. She uses 2 gallons (out of 6) for forward & backward journey from source to milepost & dropping 4 gallons in cache at milepost 1.

2.She collect 6 gallons more water at the start of 4th trip from source & drops 5 gallons at milepost 1. Now, she doesn't need to return back to source and 17 gallons of water available at milepost 1.

3.In next 2 rounds, she moves 8 gallons of water from milepost 1 to milepost 2 (1 for forward + 4 for drop + 1 for backward journey in each round). 

4.Now only 5 gallons left at milepost 2. She uses 1 gallon for journey from milepost 1 to milepost 2 and drop remaining 4 gallons at milepost 2. Now, 12 gallons of water is available at milepost 2.

3.Next, using 2 gallons (out of 6 which is maximum she can carry) she moves from milepost 2 to milepost 4 and drop 2 gallons at milepost 4 & comes back at milepost 2 using remaining 2. 

4. Again, on arriving back at milepost 2, she has left with 6 gallons of water at milepost 2 out of which she uses 2 to reach milepost 4 where 2 gallons of water still available there already collected in previous round. Now, she doesn't need to return back from
milepost 4.

5. She uses the remaining 6 gallons of water to reach at the milepost 10.

To conclude, Sara has to leave Oasis only 4 times as describe in steps 1 and 2 if she want to cross the entire 10 mile span of desert.  

Fair Distribution Of Water

In Sahara desert , 3 men found a big 24L Jar is full of water. Since there is shortage of water so they decided to distribute the water among themselves such that they all have equal amounts of it. But they only have a 13L, a 5L and an 11 liter Jar.

How do they do it? 

Here is how to do it!

Source 

Equal Distribution Of Water

What was the challenge?

1. First pour 24L into 13L and 11L jar.There will be no water in 24L jar.

2. Now pour 13L jar into 5L jar till 5L is filled. So 8L of water will be left in 13L jar.

3. This 8L of water from 13L jar is emptied in empty 24L jar.This will leave 13L jar empty.

4. Now pour 11L water from 11L jar into 13L of jar. There is still space for 2L of water in 13L jar.

5. Pour 5L jar into 13L of jar which had space for 2L only. So 3L of water will be left in 5L of jar.

6. This 3L of water is emptied in 11L jar.

7. A 13L jar full of water is again poured into 5L jar leaving behind 8L of water in it.

8. A 5L jar full of water is finally poured in 11L jar already having 3L of water. 

9. This way, 24L, 11L and 13L of jar would have 8L of water each.

Cars Across the Desert

A military car carrying an important letter must cross a desert. 

There is no petrol station on the desert and the car has space only for petrol that lasts to the middle of the desert.

There are also other cars that can transfer their petrol into one another.

How can the letter be delivered?

This is how letter can be delivered!

Source 

Delivering Letter Across The Desert

What was the task?

We need 4 such cars to deliver the letter across the desert successfully.

Let's divide the entire route into 6 parts. That means the distance that car can travel (half the total path in desert) is divided into 3 parts. To travel each part car requires 1/3rd of it's petrol in the tank.

1. At first 1/6th of total path, all cars are 2/3rd full. Now 2/3rd of the petrol from 1 car can be used to fill 1/3rd of tanks in other 2 cars (1/3 + 1/3 = 2/3). This way, we would have 2 cars full while 1 car 2/3rd full. We are leaving behind the empty car, taking 3 cars forward.

Stage 1

2. At next 1/6th of the distance, 2 full cars will use 1/3rd of their petrol hence would be 2/3rd full. And the car that was 2/3rd at previous stage would be not 1/3rd full. At this stage, the petrol from car that is 1/3rd full can be used to fill tank of 1 car completely. So we are leaving behind one another empty car here & taking fully filled car & 2/3rd filled car for next stage.

Stage 2

3. For next 1/6th of the total distance, the car that was fully filled would have 2/3rd petrol. And the car which was 2/3rd at previous stage would be now 1/3rd filled. The petrol of this car can be used to fill the tank of the first car. Now we have 1 car fully filled while other one is empty. So we can leave behind the empty car & use fully filled car for the rest half of the journey. Remember, a car which tank is full can travel half the total path.

Stage 3

 

The Coconut Problem

Ten people land on a deserted island. There they find lots of coconuts and a monkeys. During their first day they gather coconuts and put them all in a community pile. After working all day they decide to sleep and divide them into ten equal piles the next morning.

That night one castaway wakes up hungry and decides to take his share early. After dividing up the coconuts he finds he is one coconut short of ten equal piles. He also notices the monkey holding one more coconut. So he tries to take the monkey's coconut to have a total evenly divisible by 10. However when he tries to take it the monkey conks him on the head with it and kills him.

Later another castaway wakes up hungry and decides to take his share early. On the way to the coconuts he finds the body of the first castaway, which pleases him because he will now be entitled to 1/9 of the total pile. After dividing them up into nine piles he is again one coconut short and tries to take the monkey's slightly bloodied coconut. The monkey conks the second man on the head and kills him.

One by one each of the remaining castaways goes through the same process, until the 10th person to wake up gets the entire pile for himself. What is the smallest number of possible coconuts in the pile, not counting the monkeys?

Here is that smallest number! 

Source 

Number Of Coconuts In The Pile

What was the problem? 

Absolutely no need to overthink on the extra details given there. Just for a moment, we assume the number of coconuts in the community pile is divisible by 10,9,8,7,6,5,4,3,2,1.

Such a number in mathematics is called as LCM. And LCM in this case is 2520. Since each time 1 coconut was falling short of equal distribution there must be 2519 coconut in the pile initially. Let's verify the fact for all 10 distributions tried by 10 people.Each time monkey kills 1 person & number of persons among which coconuts to be distributed decreases by 1 each time.

Challenge Of Crossing Desert

Mr. Rawat wishes to cross a the Sahara desert.

It requires 6 days to cross.

One man can only carry enough food and water for 4 days.

What is the fewest number of other men required to help carry enough food for Mr. Rawat to cross ? 

He need only few helpers in the case!

Source

Efficient Way To Cross Desert

What's the challenge?

Mr. Rawat should take 2 helpers - let's name them as A & B.

Let 1 be the unit of food & water that is required for 1 day. So all are going to carry 4 units of food & water.

On the day 1, Mr. Rawat, B & A himself take the food & water from A. Then A will left with only 1 unit & to survive he should go back. In 1 day, he can consume that 1 unit of food & water & travel back to the origin.

On the day 2, Mr. Rawat & B consume 2 units food & water of helper B. Now helper B has to move back to origin with 2 units of food & water in 2 days. Again he can easily go back in 2 days covering distance traveled by him in 2 days of forward journey.

Now Mr. Rawat has 4 units of food & water which he can use in his 4 day's journey of crossing Sahara desert.