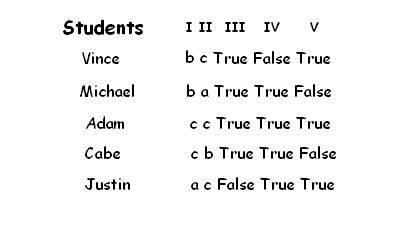
Puzzle : "Who Stole My Purse?"

An elementary school teacher had her purse stolen. The thief had to be Lilian, Judy, David, Theo, or Margaret. When questioned, each child made three statements: 

Lilian:
(1) I didn’t take the purse.
(2) I have never in my life stolen anything.
(3) Theo did it. 

Judy:
(4) I didn’t take the purse.
(5) My daddy is rich enough, and I have a purse of my own.
(6) Margaret knows who did it. 

David:
(7) I didn’t take the purse.
(8) I didn’t know Margaret before I enrolled in this school.
(9) Theo did it. 

Theo:
(10) I am not guilty.
(11) Margaret did it.
(12) Lillian is lying when she says I stole the purse. 

Margaret:
(13) I didn’t take the teacher’s purse.
(14) Judy is guilty.
(15) David can vouch for me because he has known me since I was born. 

Later, each child admitted that two of his statements were true and one was false. Assuming this is true, who stole the purse?

Here is name of the thief! 

Source

"Finally Got My Stolen Purse!"

How it was stolen?

Let's recollect all the statements given by all accused.

Lilian:
(1) I didn’t take the purse.
(2) I have never in my life stolen anything.
(3) Theo did it. 

Judy:
(4) I didn’t take the purse.
(5) My daddy is rich enough, and I have a purse of my own.
(6) Margaret knows who did it. 

David:
(7) I didn’t take the purse.
(8) I didn’t know Margaret before I enrolled in this school.
(9) Theo did it. 

Theo:
(10) I am not guilty.
(11) Margaret did it.
(12) Lillian is lying when she says I stole the purse. 

Margaret:
(13) I didn’t take the teacher’s purse.
(14) Judy is guilty.
(15) David can vouch for me because he has known me since I was born. 

Let's not forget that 2 of 3 statements made by each student are true & other is false.

Now, Theo says he is innocent in his 2 statements - (10) and (12). Since, 2 of his statements are true then (10) and (12) must be true. Hence, Theo is really innocent in case.

If Theo is innocent then both (3) and (9) are lie.

If (9) is lie, then other 2 statement of David i.e. (7) and (8) are true. If (8) is true then (15) must be lie. 

And if (15) is lie then both (13) [lie in (12) also suggests same] and (14) must be true. 

Hence, as per (14), Judy is guilty who has stolen the purse. 

Puzzle : Which one is the car thief?

A car thief, who had managed to evade the authorities in the past, unknowingly took the automobile that belonged to Inspector Detweiler. 

The sleuth wasted no time and spared no effort in discovering and carefully examining the available clues. He was able to identify four suspects with certainty that one of them was the culprit.

The four make the statements below. In total, six statements are true and six false.

Suspect A:

1. C and I have met many times before today.
2. B is guilty.
3. The car thief did not know it was the Inspector's car.

Suspect B:

1. D did not do it.
2. D's third statement is false.
3. I am innocent.

Suspect C:
 
1. I have never met A before today.
2. B is not guilty.
3. D knows how to drive.

Suspect D:
 
1. B's first statement is false.
2. I do not know how to drive.
3. A did it.

Which one is the car thief?

Know here who is that car thief? 

Solution : The Unlucky Car Thief

What was the puzzle?

Take a look at the statements made by suspects.

----------------------------------------------------------------

Suspect A:

1. C and I have met many times before today.
2. B is guilty.
3. The car thief did not know it was the Inspector's car.

Suspect B:

1. D did not do it.
2. D's third statement is false.
3. I am innocent.

Suspect C:

1. I have never met A before today.
2. B is not guilty.
3. D knows how to drive.

Suspect D:

1. B's first statement is false.
2. I do not know how to drive.
3. A did it.

----------------------------------------------------------------  

After investigation, it is found that,  in total, six statements are true and six false.

We will name statements as A1 for first statement of A, A2 for his second statement, B1 for B's first statement B2 for his second statement and so on.

1. Since, a car thief, who had managed to evade the authorities in the past, unknowingly took the automobile that belonged to Inspector Detweiler, we assume statement A3 is TRUE.

2. A1 and C1, C3 and D2 are contradicting statements. These statements are having least relevant in the process as they are not pointing to anybody else. Two of them must be TRUE and 2 must be FALSE. There are 4 TRUE and 4 FALSE statements from rest of statements.

We have, 2 FALSE statements among A1, C1, C3 and D2 for sure.

3. Assume A is a car thief. Then only A2, B2 and D3 turns out to be FALSE from rest giving in total of 5 FALSE statements only.

4. Assume C is a car thief. Then only A2, D1 and D3 are FALSE, hence total of 5 FALSE statement among all statements.

5. Assume D is a car thief. Then again only A2, B1 and D3 are FALSE, once again total 5 out of 12 given statements are FALSE in the case.

6. Assume B is a car thief. In this case, B3, C2, D1 and D3 turns out to be FALSE. Hence, total 6 out of 12 given statements are FALSE. 

This is exactly as per fact found in the investigation which suggests that exactly 6 out of 12 statements are FALSE. 

Hence, B must be a car thief. 

What day of the week is it?

A group of campers have been on vacation so long, that they've forgotten the day of the week. 

The following conversation ensues. 

Darryl: "What's the day? I don't think it is Thursday, Friday or Saturday." 

Tracy: "Well that doesn't narrow it down much. Yesterday was Sunday." 

Melissa: "Yesterday wasn't Sunday, tomorrow is Sunday." 

Ben: "The day after tomorrow is Saturday." 

Adrienne: "The day before yesterday was Thursday." 

Susie: "Tomorrow is Saturday." 

David: "I know that the day after tomorrow is not Friday." 

If only one person's statement is true, what day of the week is it?

So it was THIS day of the week!

The Forgotten Day of Week is Wednesday!

What was the puzzle?

Let's see once again the conversation that campers had - 

--------------------------------------------------------


Darryl: "What's the day? I don't think it is Thursday, Friday or Saturday." 

Tracy: "Well that doesn't narrow it down much. Yesterday was Sunday." 

Melissa: "Yesterday wasn't Sunday, tomorrow is Sunday." 

Ben: "The day after tomorrow is Saturday." 

Adrienne: "The day before yesterday was Thursday." 

Susie: "Tomorrow is Saturday." 

David: "I know that the day after tomorrow is not Friday."

-----------------------------------------------------

Let's see what day statement of each is suggesting -

Darryl - Sunday, Monday, Tuesday, Wednesday.

Tracy - Monday.

Melissa - Saturday.

Ben - Thursday.

Adrienne - Saturday.

Susie - Friday.

David - Sunday, Monday, Tuesday, Thursday, Friday or Saturday.  

If we assume David's statement is TRUE then one of statements of Darryl (Sunday, Monday, Tuesday are common) or Tracy (Monday is common) or Melissa & Adrienne (Saturday is common) or Susie (Friday is common) or Ben (Thursday is common) has to be also TRUE. But this is against the given data that only 1 of the statement is TRUE.

Hence, David's statement must be FALSE and the only day that isn't pointed by David is Wednesday.

So the day must be Wednesday as suggested correctly by Darryl and thereby making statements of every other camper including David FALSE. 

"Are you holding true or fake coin?"

You have 101 coins, and you know that 50 of them are counterfeit. Every true coin has the same weight, an unknown integer, and every false coin has the same weight,which differs from that of a true coin by 1 gram. You also have a two-pan pointer scale that will show you the difference in weight between the contents of each pan. You choose one coin. 

Can you tell in a single weighing whether it’s true or false?

Well, this trick will help you to identify that coin! 

Knowing The Truth of the Coin in Hand!

What was the task given?

Yes, you can tell that whether the coin is true or false with single weighting.Just divide 100 coins into 2 groups of 50 coins each & put into 2 pans of weighing balance.

Let's assume true coin weighs 1 gram (or 2 gram) & fake coin weighs 2 gram (or 1 gram). Remember, if the sum of 2 integers is even then difference between two is bound to be even. And if the sum of those is odd then difference between them has to be odd.

CASE 1 :

If the coin that you are holding is true then the total weight on the balance will be
50 + (50x2) = 50 + 100 = 150  (or 50x2 + 50 = 150). So, the total sum of weights in 2 pans is even, hence difference between them has to be even. For example, if those 150 grams are distributed as 80 vs 70 then difference between them is 10 which is even.


CASE 2 :

If the coin you are holding is fake then the total weight on the balance will be
51 + (49x2) = 51 + 98 = 149 (or 51x2 + 49x1 = 153).

Here, total is odd hence the difference must be odd too. For example, if above 149 grams are distributed as 90 vs 59 then pointer of balance will point at 31 which is odd.

Conclusion:

In short, you have to notice the difference between 2 weights on the pans. If it's even then the coin you are holding is true and if difference is odd then you are holding a fake coin.
 

Fill in the Empty Boxes

Is it possible to fill each box in with an arithmetic operation so that this becomes a true equation?

Did you too find it true? 

Correct Operators in Empty Boxes!

What wasn't looking possible?

Yes, it's possible. All you need to do is recall BODMAS (Brackets, Of, Division, Multiplication, Addition, Subtraction) rule in mathematics that we learned in school.

Help The Policeman in Finding The Culprit

Late one evening, a car ran over a pedestrian in a narrow bystreet and drove away without stopping. A policeman who saw the vehicle leave the scene of the accident reported it moving at very high speed. The accident itself was witnessed by six bystanders. They provided the following conflicting accounts of what had happened:

• It was a blue car, driven by a man;
• The car was moving at high speed, its headlights were turned off;
• The car did have license plates, it wasn’t going very fast;
• It was a Toyota, it’s headlights were turned off;
• The car didn’t have license plates, the driver was a woman;
• It was a gray Ford.

When the the car and its driver were finally apprehended, it turned out that only one of the six eyewitnesses gave a fully correct description. Each of the other five provided one true and one false piece of information.

Keeping that in mind, can you determine the following:

— What was the car’s brand?

— What color was the car?

— Was the car going fast or slow?

— Did it have license plates?

— Were its headlights turned on?

— Was the driver a man or a woman? 

Read all the answers here!

To Help The Policeman in Finding The Culprit

But why he needs help? 

Let's recollect all the statements made by all 6 bystanders.

 1.It was a blue car, driven by a man.

 2.The car was moving at high speed, its headlights were turned off.

 3.The car did have license plates, it wasn’t going very fast.

 4.It was a Toyota, it’s headlights were turned off.

 5.The car didn’t have license plates, the driver was a woman.

 6 It was a gray Ford (It was gray car; it was Ford).

-----------------------------------------------------------------------------------

If we believe in report made by Policeman where he stated that the car was moving at very high speed; then the part of the Statement 3 made by third bystander where he says car wasn't going fast turns out to be false. Hence, other part of his statement must be true. So the car must have license plates.

If the car has license plates; then 1st part of the Statement 5 will be false & other part must be true. Hence, the driver must be a woman.

If the driver was a woman, then 2nd part of the Statement 1 turns false making part 1 to be true. Hence, the color of the car must be blue.

If the car was at high speed then the entire Statement 2 must be true or it's 2nd part must be false.

Let's assume 2nd part of the statement 2 be false. Then 2nd part of statement 4 also must be false leaving 1st part to be true. That means the car was Toyota. But this makes statement 6 entirely false (as we already know color of car is blue). This contradicts the crucial data given - Each of the other five provided one true and one false piece of information. In the case, there will be no eyewitness giving full correct description.

So the entire Statement 2 must be true. Hence, the car was with it's headlight off.

If headlights were turned off then 2nd part of the Statement 4 must be true and 1st part false. That means, car wasn't Toyota.

And if car wasn't Toyota, as per Statement 6, it must be Ford but not of gray color.
This matches our early conclusion where we concludes color of the car was blue.

Conclusions:

1.What was the car’s brand?

   - Ford

2.What color was the car?

   - Blue

3.Was the car going fast or slow?

   - Fast

4.Did it have license plates?

   - Yes, it had.

 

5.Were its headlights turned on?

   - No, those were off.

6.Was the driver a man or a woman?

   - A woman. 

Test of an Examiner

Five students - Adam, Cabe, Justin, Michael and Vince appeared for a competitive exam. There were total five questions asked from them from which were two multiple choice questions (a, b or c) and three were true/false questions. Their answers are given as follows:

Name I II III IV V

Cabe c b True True False

Adam c c True True True

Justin a c False True True

Michael b a True True False

Vince b c True False True

Also, no two students got the same number of correct answers. Can you tell the correct answer? Also, what are their individual score?

Know correct answers and scores here!

Source 

Responding To Test of an Examiner

What was the test?

There are 2 possibilities of scores & that are either 0,1,2,3,4 or 1,2,3,4,5. First of all, let's arrange students' responses in order like below.

Table 1

What we notice here is that, there are few responses to same question by different student matching.

For the Question III, only Justin given different answers than other.

Case 1 : If we assume Justin's answer is correct then rest of all are wrong in response to Question III. That means either maximum score in test is 4 or Justin himself has scored 1 to 5.

Let's test that apart from Justin who can have score of 4. If any body other scores 4 then he must share at least 3 similar answers with other (excluding Answer III; refer image below). Only Adam has exact 3 matching responses with Justin.

Table 2

If Adam's score is 4 (Answers to I, II, IV, V are correct) then, Justin too would score 4 (Answers to II, III, IV,V are correct) since Adam & Justin have same responses to Questions II, IV,V).
  
If nobody scoring as 4 then Justin can have score of 4 or 5.

Case 1.1 : If his score is 4 then there has to be somebody has to be there scoring 0. Now Vince and Adam has at least 2 responses matching with the Justin. That means they can't score 0 since even 1 answer is wrong as Justin the other must be correct as Justin. Michael or Cabe can have 0 score in the case. If anybody of them has score 0 then answer as a TRUE to the Question IV is incorrect i.e. correct Answer IV is FALSE. So Justin is WRONG in Answer IV only. In short, a, c, FALSE, FALSE, TRUE is correct combination of answers. But thing is here in the case both Michael and Cabe would have score 0! Hence Justin's score can't be 4 too.

Case 1.2 : If Justin's score is 5, then a, c, FALSE, TRUE, TRUE are the right answers. No one would score 4 in that case with 3 as second highest by Adam.

Wrong Looking Correct Mathematical Equation!

The following question it puts forth you:

25 - 55 + (85 + 65) = ?

Then, you are told that even though you might think its wrong, the correct answer is actually 5!

Whats your reaction to it? How can this be true? 

 That's how it's perfectly correct!

That's How Equation is Correct!

Why it was looking wrong? 

If you read the data carefully then you will notice '!' attached to number 5 which is being claimed answer. Actually claimed answer is 5! not 5 Read it again...

"Then, you are told that even though you might think its wrong, the correct answer is actually 5!."

Now use of '!' is not limited to the sentences only. In mathematics it's a 'factorial'.

So 5! = 5 x 4 x 3 x 2 x 1 = 120 and 25 - 55 + (85 + 65) = 120 and hence,

25 - 55 + (85 + 65) = 5! 

Now doesn't it look the correct equation? 

The CryptArithmetic Problem

Can you solve the below alphametic riddle by replacing letters of words by a numbers so that the below equation holds true?

BASE +
BALL
---------
GAMES
----------

 
Find numbers replaced letters here! 

Source 

The CryptArithmetic Problem's Solution

What was the problem?

Let's first recall the given equation.

  BASE +
  BALL
---------
GAMES
----------

We are assuming repeating the numbers are not allowed. 

Let's first take last 2 digits operation into consideration i.e. SE + LL = ES or 1ES (carry in 2 digit operation can't exceed 1). For a moment, let's assume no carry generated.

10S + E + 10L + L = 10E + S .....(1)

9 (E - S) = 11L

To satisfy this equation L must be 9 and (E - S) must be equal to 11. But difference between 2 digits can't exceed 9. Hence, SE + LL must have generated carry.So rewriting (1),

10S + E + 10L + L = 100 + 10E + S

9 (E - S) + 100 = 11L

Now if [9 (E - S)] exceeds 99 then L must be greater than 9. But L must be digit from 0 to 9. Hence, [9 (E - S)] must be negative bringing down LHS below 100. Only value of E - S to satisfy the given condition is -5 with L = 5. Or we can say, S - E = 5.

Now, possible pairs for SE are (9,4), (8,3), (7,2), (6,1), (5,0). Out of these only (8,3) is pair that makes equation SE + LL = SE + 55 = 1ES i.e. 83 + 55 = 138. Hence, S = 8 and E = 3.

Replacing letters with numbers that we have got so far.

    1---------
  BA83 +
  BA55
---------
GAM38
----------

Now, M = 2A + 1. Hence, M must be odd number that could be any one among 1,7,9 (since 3 and 5 already used for E and L respectively).

If M = 1, then A = 0 and B must be 5. But L = 5 hence M can't be 1

If M = 7, then A = 3 or A = 8. If A = 3 then B = 1.5 and that's not valid digit. And if A = 8 then it generates carry 1 and 2B + 1 = 8 again leaves B = 3.5 - not a perfect digit.

If M = 9, then A = 4 (A = 9 not possible as M = 9) and B must be 7 with carry G = 1.Hence for first 2 digits we have 74 + 74 + 1 = 149.

Finally, rewriting the entire equation with numbers replacing digits as -

    1
---------
  7483 +
  7455
---------
14938
----------

So numbers for letters are S = 8, E = 3, L = 5, A = 4, B = 7, M = 9 and G = 1.