Posts
Cracking of The Code in Steps...
- Get link
- X
- Other Apps
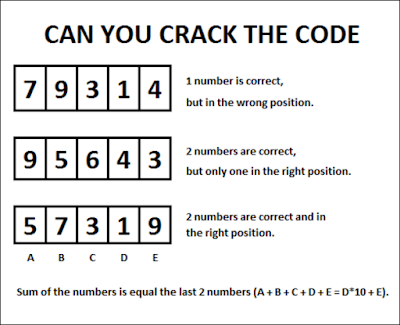
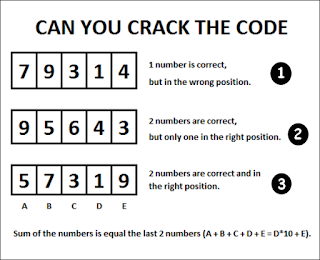
What was the challenge?
Let's number the clues as 1, 2, 3.
Now following step by step process here onward.
1. The numbers 3 & 1 are common in first & third combinations. Now both must not be the part of original number as in that case Clue 1 will be invalid.
2. If numbers 3 & 1 are not correct in third combination then the correct 2 numbers must be among 5,7,9.
3. But it can't be both 7 and 9 as again that would make clue 1 invalid! Hence, the 5 is part of the original key & in correct position as in third combination. So we have got first digit of key as a 5.
4. The 1 correct number in clue 2 is 5 & that's in wrong position. If other is assumed to be 9 & to be in right position then it contradict the clue 3. So the second digit must be 7.
5.The only correct number in clue 1 is 7 & that's in wrong position. That means numbers 1,3,4,9 must not be the part of the code.
6. Since 3,4,9 eliminated in previous steps, the only number that is correct and in right position must be 6 in suggestion made by clue 2. So far we have got 3 digits of the code as 576XX.
7. Last 2 digits can be any combination from 0,2,5,7,6,8. Now addition of all digits is equal to the number formed by last 2 digits. It's impossible that the addition of all digits exceeds 50. Hence, the second last digit must be 2.
8. Now both 57620 or 57628 are perfectly valid where sum of all digits equals to number formed by last 2 digits.
Fill In Next Three Blanks
- Get link
- X
- Other Apps
Can you find the next three numbers in the given series?
4, 6, 12, 18, 30, 42, 60, 72, 102, 108,_,_,_
Escape to the answer!
Source
4, 6, 12, 18, 30, 42, 60, 72, 102, 108,_,_,_
Escape to the answer!
Source
Numbers Deserving Blanks
- Get link
- X
- Other Apps
How was series looking?
Let's take a look at the series once again.
4, 6, 12, 18, 30, 42, 60, 72, 102, 108,_,_,_
If we carefully observe the series, we can find that every number is in between 2 PRIME numbers.
4 is in between 3 and 5.
6 is in between 5 and 7.
12 is in between 11 and 13.
So on........
108 is in between 107 and 109.
Hence next numbers should be 138, 150 and 180!
Mixed up Apples at the Farm
- Get link
- X
- Other Apps
Someone has mixed up the apples. Read carefully what has happened. Can you find a way to help solve the problem.
• There are 10 baskets containing apples.
• There are various amounts of apples in each basket ranging from 10 to 20.
• 9 of the baskets contain apples weighing 4 ounces each.
• 1 of the baskets contains apples weighing 5 ounces each.
• All the apples look the same.
• The equipment you have is a set of scales and an empty basket.
• It is late and the truck is waiting to take the apples to market. You only have time to make one measurement using the scales.
Take out the basket contains apples weighing 5 ounces each.
Here is how you can identify that basket!
Source
• There are 10 baskets containing apples.
• There are various amounts of apples in each basket ranging from 10 to 20.
• 9 of the baskets contain apples weighing 4 ounces each.
• 1 of the baskets contains apples weighing 5 ounces each.
• All the apples look the same.
• The equipment you have is a set of scales and an empty basket.
• It is late and the truck is waiting to take the apples to market. You only have time to make one measurement using the scales.
Take out the basket contains apples weighing 5 ounces each.
Here is how you can identify that basket!
Source
Sorting of Mixed Up Apples
- Get link
- X
- Other Apps
How they were mixed?
Let's number all the baskets from 1 to 10. Just take out 1 apple from first basket, 2 from second, 3 from third & so on. We would have 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10 = 55 apples now. If each of them was of 4 oz, then these 55 apples together would have weighed as 55 x 4 = 220 oz. Since 1 basket have apples each weighing 5 oz, these 55 apples would weigh more than 220 oz.
Let's say it weighs 222 oz, then the 2 apples taken by second basket must be of 5 oz each. And if it weighs 228 then there are 8 apples weighing more than 4 oz (i.e. 5 oz each). Hence that eight basket must have all apples weighing.
So depending on how much weight of 55 apples exceeds 220 oz, we can identify the basket with apples weighing 5 oz each.
The CryptArithmetic Problem
- Get link
- X
- Other Apps
Can you solve the below alphametic riddle by replacing letters of words by a numbers so that the below equation holds true?
BASE +
BALL
---------
GAMES
----------
Find numbers replaced letters here!
Source
BASE +
BALL
---------
GAMES
----------
Find numbers replaced letters here!
Source