Bags of Marbles - Puzzle

There are three bags, each containing two marbles. Bag A contains two white marbles, Bag B contains two black marbles, and Bag C contains one white marble and one black marble. 

You pick a random bag and take out one marble, which is white. 

What is the probability that the remaining marble from the same bag is also white?

Here is the solution! 

Bags of Marbles Puzzle - Solution

What is the puzzle?

Well, the probability that the second marble is also white should be 1/2 not 2/3 as most of answers to this puzzle claims!

There is no way that you have picked up the bag B having 2 black marbles since the first marble you have drawn is white. Hence, you must have picked up Bag A or C.

Claim :  

You chose Bag A, first white marble. The other marble will be white

You chose Bag A, second white marble. The other marble will be white

You chose Bag C, the white marble. The other marble will be black

So 2 out of 3 possibilities are white.


Hence, 2/3 is the probability of the second ball is also white.


Correct Approach :

The probability of the particular bag being picked randomly is 1/3 initially. Once the bag picked and since the marble drawn from it is white, the bag must be either A (2 whites) or C (1 white, 1 black).

The probability that the bag you picked is A (or C) must be 1/2 and hence the probability that second marble is also white (or not white) has to be also 1/2.

The Last Bean in the Pot

A pot contains 75 white beans and 150 black ones. Next to the pot is a large pile of black beans. 

A somewhat demented cook removes the beans from the pot, one at a time, according to the following strange rule: 

He removes two beans from the pot at random. If at least one of the beans is black, he places it on the bean-pile and drops the other bean, no matter what color, back in the pot. If both beans are white, on the other hand, he discards both of them and removes one black bean from the pile and drops it in the pot.
 
At each turn of this procedure, the pot has one less bean in it. Eventually, just one bean is left in the pot. What color is it?

And the color of last bean is...... 

The Color of Last Bean in the Pot

Little story behind the title!

There are 75 WHITE beans in the pot i.e. they are odd in numbers. Since, they are always taken out in pair, in the end there will be 1 WHITE bean left out.

At some point, when there are 3 bean are left in the pot then there has to be 2 BLACK and 1 WHITE beans in the pot. They can't be 1 BLACK and 2 WHITE beans as for that 73 WHITE beans need to be taken out which is not possible since WHITE are always taken in pair.

So if cook pick 2 BLACK beans (or BLACK & WHITE) at this point then anyhow BLACK and WHITE will be left in the pot. Now, when he pick this pair of BLACK and WHITE then he puts BLACK on the pile and drop WHITE back to the pot as per his rule.

Eventually, WHITE bean will be left in the pot.

"Spot on the Forehead" Sequel Contest

After losing the "Spot on the Forehead" contest, the two defeated Puzzle Masters complained that the winner had made a slight pause before raising his hand, thus derailing their deductive reasoning train of thought. 

And so the Grand Master vowed to set up a truly fair test to reveal the best logician among them.


He showed the three men 5 hats - two white and three black. 

Then he turned off the lights in the room and put a hat on each Puzzle Master's head. After that the old sage hid the remaining two hats, but before he could turn the lights on, one of the Masters, as chance would have it, the winner of the previous contest, announced the color of his hat. 

And he was right once again.

What color was his hat? What could have been his reasoning? 

The winner is wisest for a reason! 

Master of Logic For a Reason!

How the master was challenged?

Let's assume once again A, B and C are those logicians and C has guessed the color of own hat correctly. Here is what he must have thought - 

============================================================================

"I'm assuming the grand master is conducting this test fairly denying any sort of advantage to any participant.

With that assumption, the grand master can't put 2 white and 1 black hat on heads. In that case, the person having black hat and watching 2 white hats on others' head would know the color of own hat immediately.

For fair play, he can't put 2 blacks and 1 white hat either. That will give unfair advantage to the logicians wearing black hats. Suppose A and B are wearing black and I'm wearing white hat. Now, what A (or B) would be thinking - 

         ---------------------------------------------------------------------------------------------------

       " I'm A (or B) and I can see 1 black and 1 white hat (on head of C). If I have white 
         hat on my head then B (or A) would know color of his hat as black as there are 
         only 2 white hats available and those would be on my head and C's head.

         Moreover, 1 black and 2 white hats already eliminated as it's unfair distribution.

         That means I must be wearing black hat."

         ---------------------------------------------------------------------------------------------------

That's how the combination of 2 black and 1 white hats also eliminated from fair play.

Hence, all of three must be wearing black hats is only fair distribution giving all of us equal chance of winning and hence I must be wearing black hat only. 

============================================================================ 

Note : Here, C is assumed as a winner for only sake of convenience, otherwise either A or B whoever is wisest can be winner.

Maximize The Chance of White Ball

There are two empty bowls in a room. You have 50 white balls and 50 black balls. After you place the balls in the bowls, a random ball will be picked from a random bowl. Distribute the balls (all of them) into the bowls to maximize the chance of picking a white ball. 

This is the way to maximize the chances!

Way to Maximize White Ball Probability

What was the task given?

Let's distribute 50 black ball in one bowl & other 50 white ball in another bowl.

Then,

Probability (White Ball) = (1/2)(0/50) + (1/2)(50/50) = 0.5.

Now, if 1 white ball is kept in 1 bowl and other 49 white + 50 black = 99 balls in other bowl, then


Probability (White Ball) = (1/2)(1/1) + (1/2)(49/99) = 0.747.

That's nearly equal to 3/4 which is certainly higher than the previous case. And that's the way of maximizing the probability of white ball.

What is Color of His Hat?

There is a basket full of hats. 3 of them are white and 2 of them are black. There are 3 men Tom, Tim, and Jim. They each take a hat out of the basket and put it on their heads without seeing the hat they selected or the hats the other men selected.

The men arrange themselves so Tom can see Tim and Jim’s hats, Tim can see Jim’s hat, and Jim can’t see anyone’s hat.

Tom is asked what color his hat is and he says he doesn’t know.

Tim is asked the same question, and he also doesn’t know.

Finally, Jim is asked the question, and he does know.

What color is his hat?

Know color of his hat! 

'THIS' is The Color of His Hat!

What was the challenge?

Since there are only 2 black hats if Tom had saw 2 black hats (on heads of Tim & Jim) then he would have realized that he must be wearing white hat. Since, he says he doesn't know color of his hat that mean he can see either 2 white  hats or 1 black & 1 white hat on heads of other 2.

Now when Tim is saying he doesn't know color of his hat that means Jim must not be wearing black hat. If Jim had black hat then Tim would know that his color of hat is white but can't be black again as in that case Tom would have identified color of his hat in first attempt.

Hence, Jim must be wearing white hat! 

Mathematical Puzzle On The Chess Board

A white rook and a black bishop of a standard chess set are randomly placed on a chessboard

What is the probability that one is attacking the other?


You can skip to the answer! 
  

Resolving Mathematical Puzzle On Chess Board

But what was the puzzle?

Let's recall the definition of the probability. It's ratio of number of desired combinations to the number of total possible combinations.

A Rook and Bishop can have 64 Permutations 2 = 64 X 63 = 4032 possible combinations on a standard chess board if placed randomly.

Now there are 2 possible cases - The Rook attacking Bishop and the Bishop attacking Rook. Both can't attack each other simultaneously.

CASE 1 : The Rook attacking the Bishop 

The Rook can have 64 possible positions on the chess board and for every position it attacks 14 other position in it's attacking lines. That means 64 X 14 = 896 possible combinations where Rook is attacking Bishop. 

 CASE 2 : The Bishop attacking the Rook

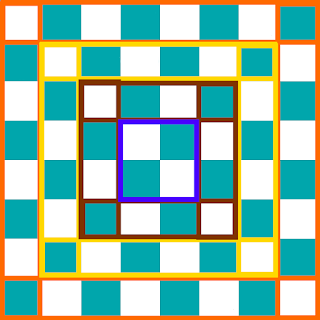
Now imagine 4 hallow co-centrist squares around the center of the chess board with outermost have side 8 units & innermost having side 2 units.(See below)

Here each square has side with thickness of 1 unit.

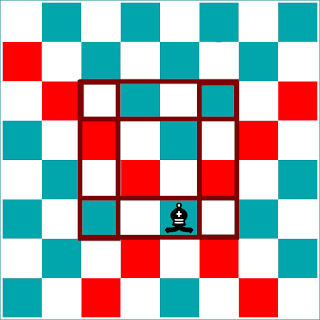
If the Bishop is anywhere on outermost square which has 28 possible positions then it attack 7 other positions.( See below pics).

So there are 28 x 7 = 196 possible combinations where Bishop attacking the Rook.

--------------------------------------------------------------------------

Now if the bishop is anywhere on 20 squares of inner square then 9 other positions will be in it's attacking lines. (See below).

  

In this way, there will be 20 X 9 = 180 such combinations where the bishop will attack the rook. 

--------------------------------------------------------------------------

Now if the Bishop is placed anywhere on the 12 squares of more inner square then 11 other positions will be in it's lines of attack.( See below).

In short, there are 12 X 11 = 132 combination where Bishop will be attacking the rook.

--------------------------------------------------------------------------

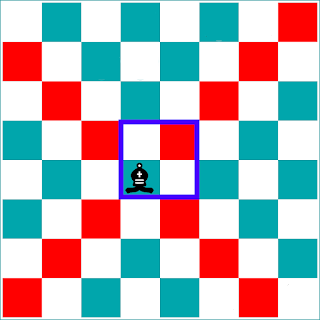
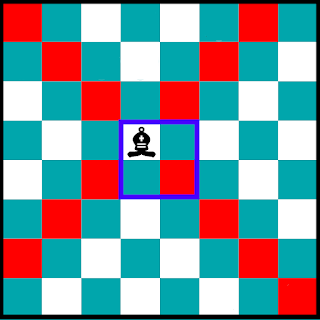
And finally, if the bishop is placed at any of 4 positions of the innermost square then it will attack 13 other positions like below.

That is, there will be 4 X 13 = 52  such combinations where the Bishop will be attacking the Rook.

-------------------------------------------------------------------------------

Adding all possible combinations of CASE 1 and CASE 2 as - 896 + 196 + 180 + 132 + 52 =  1456. It means there are 1456 possible combinations where either the Rook attacking Bishop or Bishop attacking the Rook.

The Required Probability  = Number of Required Combinations / Number of Total Combinations = 1456 / 4032 = 0.3611

To conclude, 0.3611 is the probability that the Rook or Bishop attacking each other if place randomly on standard chess board.

NOTE : Don't get confused with black Bishop on black square used in illustrations of attacking lines in CASE 2. Even if it was black Bishop on white square then also it would attack same other positions mentioned in that particular consideration. And random placement means it could be anything - on black or on white.

"Your Surname Tells My Shirt's Color!"

Mr. Yellow, Mr. Black and Mr. Brown, three best friends since kindergarten meet in a function after 5 years. The three of them are wearing either a Yellow, Black or Brown shirt.
After giving each other a friendly hug, Mr. Black says, "Hey, did you notice that we are wearing a different colored shirt than our names!"
The man wearing a Brown shirt said, "Wow I certainly did not notice that but you are right Mr. Black."
Based on this conversation, can you find our who was wearing which colored shirt? 

 
Know color of each person's shirt here! 
  

Surnames And Shuffling of Shirts

Read this conversation first!

One thing is sure that Mr.Black is not wearing the BLACK shirt. He is also not wearing BROWN as well as we found the other person wearing the BROWN colored shirt is agreed with the statement made by Mr.BLACK in a conversation. So only color left is the YELLOW & Mr. Black must be wearing that.

Now, the colors left are BROWN and BLACK and persons left are Mr. Yellow and Mr.Brown. Since, each of them wearing different color than his surname, Mr. Brown must be wearing the BLACK shirt. And hence, Mr. Yellow is wearing the BROWN shirt! 

To conclude, Mr.Black is wearing YELLOW shirt, Mr. Brown is wearing BLACK shirt & Mr. Yellow must be wearing BROWN shirt.

 

Black or White Dot on Forehead

There are five men, let's say Tarun, Harish, Lavesh, Manoj and Manish. All of them have a dot mark in their forehead. They can't see the dot on their own forehead, but can see the ones on others. The owner of WHITE dot is an honest person and will never lie, while the owner of BLACK dot always tell the lie.

This are the statement from Tarun, Harish, Lavesh, and Manish:

Tarun: 'I see 3 whites and 1 black'

Harish: 'I see 4 black'

Lavesh: 'I see 3 black and 1 white'

Manish: 'I see 4 white'

What color is the dot on each Tarun, Harish, Lavesh, Manoj, Manish forehead?

Know color of dot on each forehead!

Source 

Men with White Dots on Foreheads

Read this story first!

Let's take a look at who said what. 

Tarun: 'I see 3 whites and 1 black'
Harish: 'I see 4 black'
Lavesh: 'I see 3 black and 1 white'
Manish: 'I see 4 white'
 
Harish and Manish made very contracting statements; so are statement of Tarun and Lavesh. That means, only one of them is telling the truth. Hence, only one of 4 have WHITE dot on his forehead. Till now we don't have idea of what Manoj had on his forehead. Even if he has WHITE dot then there can be maximum 2 WHITE dots among those 5.    

Truth of Tarun and Manish:

Tarun and Manish must be lying as they are saying that they have seen 3 and 4 WHITE dots respectively. According to our first conclusion, there can be maximum 2 WHITE dots possible.

Truth of Harish:

Next if we assume Harish has WHITE dot and telling the truth then all other must be lying including Manoj and Lavesh. As per Lavesh, he had seen 3 BLACK and 1 WHITE dot. Now he must have seen BLACK dots on foreheads of Manoj, Tarun, Manish and WHITE dot on forehead of Harish as assumed. That mean he is telling truth though he has BLACK dot. But the man with BLACK is always lying, hence this case is also INVALID. 

Truth of Lavesh:

So the only person left is Lavesh and must be telling the TRUTH with WHITE dot on his forehead. Hence, as his statement is suggesting, the Manoj must have WHITE dot and other three Tarun, Lavesh, Harish have BLACK dots (we already concluded these 3 are lying). 

Time Taken For The Journey

A RED ant is sitting on one side of a table (point X) and a BLACK ant is sitting on the opposite side of the table (point Y).

Now both of them decides to exchange their places and starts crawling. On the way, both of the ants meet and after that, it takes 20 seconds for the RED ant to move to point Y and it takes 5 seconds for the BLACK ant to reach point X.

Find out the total time taken by the RED and the BLACK ant to make the journey.

Here are steps to calculate! 

Source 

Calculation of Time For The Journey

What was the journey?

Let the speed of RED ant is R & that of BLACK ant is B

Let time taken by them to meet be T.

Now we will apply the basic formula of distance:

Distance = Speed * Time.

The RED ant travels R T distance before meeting and 20 R after the meeting.

The BLACK ant travels B T distance before meeting and 5 B after the meeting.

Now as per the question,The distance traveled by RED ant before they both meet will be equal to the distance covered by BLACK ant after they meet. We can say the same for the vice versa case as well.

Thus,

RT = 5B and BT = 20R i.e. B = 20R/T, putting in RT = 5B

R T = [20R/T] * 5

RT = 100R/T

T^2 = 100

T = 10.

Thus the RED ant will require 10 + 20 = 30 seconds to travel the distance.

And the BLACK ant will take 10 + 5 = 15 seconds to travel the distance. 

Who Will Shout First?

4 criminals are caught and are to be punished. The Judge allows them to be freed if they can solve a puzzle. If they do not, they will be hung. They agreed.
The 4 criminals are lined up on some steps (shown in picture). They are all facing in the same direction. A wall separates the fourth man from the other three.
So to summarize :-

Man 1 can see men 2 and 3.

Man 2 can see man 3.

Man 3 can see none of the others.

Man 4 can see none of the others.

The criminals are wearing hats. They are told that there are two white hats and two black hats. The men initially don't know what color hat they are wearing. They are told to shout out the color of the hat that they are wearing as soon as they know for certain what color it is.

They are not allowed to turn round or move.

They are not allowed to talk to each other.

They are not allowed to take their hats off.

Who is the first person to shout out and why?

Who will shout first?

Know that person here! 

Source 

Chance of Knowing Color of Hat

Here is the given situation! 

Well, there are 2 possibilities of answer depending on what combination of hats Man 2 & Man 3 are wearing. One thing is sure Man 4 has least chance for getting what he is wearing. His crime must be the most serious one!

Case 1 : If Man 2 & Man 3 wearing same color of hat.

In this case, Man 1 would immediately come to know that he is wearing opposite color of hat & would shout out. In simple words, if Man 2 & Man 3 wearing WHITE (or BLACK) hats, then Man 1 & Man 4 must be wearing BLACK (or WHITE) hats. Since there is no chance for Man 4 to see Man 2 & Man 3 but Man 1 could see Man 2 & Man 3, he must be first to know color of hat he is wearing.


Case 2 : If Man 2 & Man 3 wearing hats of different color.

Now here Man 2 has to be very intelligent (like the reader as he has chosen to read this further). In this case, there is no way for Man 1 to come at any conclusion as there might be WHITE or BLACK hat on his head. After waiting for a while Man 2 has to apply some logic & should think this in his mind.

'Since Man 1 is not shouting he must be unsure with color of his hat. He would be sure only in case if I & Man 3 wearing same color of hats. Since he is unsure; the color of my hat must be opposite of Man 3's hat.' 

Now the judge if he is honest would put criminal with least serious crime at number 1 (or  & 2) same color (or different) hats on Man 2 & Man 3.